With this service, you can find the largest and smallest value of a function one variable f(x) with the design of the solution in Word. If the function f(x,y) is given, therefore, it is necessary to find the extremum of the function of two variables . You can also find the intervals of increase and decrease of the function.

Function entry rules:

A necessary condition for an extremum of a function of one variable

The equation f "0 (x *) \u003d 0 is a necessary condition for the extremum of a function of one variable, i.e. at the point x * the first derivative of the function must vanish. It selects stationary points x c at which the function does not increase and does not decrease .

A sufficient condition for an extremum of a function of one variable

Let f 0 (x) be twice differentiable with respect to x belonging to the set D . If at the point x * the condition is met:

F" 0 (x *) = 0
f"" 0 (x *) > 0

Then the point x * is the point of the local (global) minimum of the function.

If at the point x * the condition is met:

F" 0 (x *) = 0
f"" 0 (x *)< 0

That point x * is a local (global) maximum.

Example #1. Find the largest and smallest values ​​of the function: on the segment .
Solution.

The critical point is one x 1 = 2 (f'(x)=0). This point belongs to the segment . (The point x=0 is not critical, since 0∉).
We calculate the values ​​of the function at the ends of the segment and at the critical point.
f(1)=9, f(2)= 5 / 2 , f(3)=3 8 / 81
Answer: f min = 5 / 2 for x=2; f max =9 at x=1

Example #2. Using higher order derivatives, find the extremum of the function y=x-2sin(x) .
Solution.
Find the derivative of the function: y’=1-2cos(x) . Let us find the critical points: 1-cos(x)=2, cos(x)=1, x=± π / 3 +2πk, k∈Z. We find y''=2sin(x), calculate , so x= π / 3 +2πk, k∈Z are the minimum points of the function; , so x=- π / 3 +2πk, k∈Z are the maximum points of the function.

Example #3. Investigate the extremum function in the neighborhood of the point x=0.
Solution. Here it is necessary to find the extrema of the function. If the extremum x=0 , then find out its type (minimum or maximum). If among the found points there is no x = 0, then calculate the value of the function f(x=0).
It should be noted that when the derivative on each side of a given point does not change its sign, the possible situations are not exhausted even for differentiable functions: it may happen that for an arbitrarily small neighborhood on one side of the point x 0 or on both sides, the derivative changes sign. At these points, one has to apply other methods to study functions to an extremum.

Example #4. Divide the number 49 into two terms, the product of which will be the largest.
Solution. Let x be the first term. Then (49-x) is the second term.
The product will be maximal: x (49-x) → max
or
49x-x2

Largest cylinder volume

Find the dimensions of the cylinder of the largest volume, made from a workpiece in the form of a ball of radius R.
Solution:

The volume of the cylinder is: V = πr 2 H
where H = 2h,
Substitute these values ​​into the objective function.

V → max
Find the extremum of the function. Since the volume function V(h) depends only on one variable, we will find the derivative using the service

From this article, the reader will learn about what an extremum of functional value is, as well as about the features of its use in practice. The study of such a concept is extremely important for understanding the foundations of higher mathematics. This topic is fundamental to a deeper study of the course.

In contact with

What is an extreme?

In the school course, many definitions of the concept of "extremum" are given. This article is intended to give the deepest and clearest understanding of the term for those who are ignorant of the issue. So, the term is understood to what extent the functional interval acquires a minimum or maximum value on a particular set.

The extremum is both the minimum value of the function and the maximum at the same time. There is a minimum point and a maximum point, that is, the extreme values ​​of the argument on the graph. The main sciences in which this concept is used:

  • statistics;
  • machine control;
  • econometrics.

Extreme points play an important role in determining the sequence of a given function. The coordinate system on the graph at its best shows the change in extreme position depending on the change in functionality.

Extrema of the derivative function

There is also such a thing as a "derivative". It is necessary to determine the extremum point. It is important not to confuse the minimum or maximum points with the largest and smallest values. These are different concepts, although they may seem similar.

The value of the function is the main factor in determining how to find the maximum point. The derivative is not formed from the values, but exclusively from its extreme position in one order or another.

The derivative itself is determined based on the data of the extreme points, and not the largest or smallest value. In Russian schools, the line between these two concepts is not clearly drawn, which affects the understanding of this topic in general.

Let's now consider such a thing as a "sharp extremum". To date, there is an acute minimum value and an acute maximum value. The definition is given in accordance with the Russian classification of critical points of a function. The concept of an extremum point is the basis for finding critical points on a chart.

To define such a concept, Fermat's theorem is used. It is the most important in the study of extreme points and gives a clear idea of ​​their existence in one form or another. To ensure extremeness, it is important to create certain conditions for decreasing or increasing on the chart.

To accurately answer the question "how to find the maximum point", you must follow these provisions:

  1. Finding the exact area of ​​definition on the chart.
  2. Search for the derivative of a function and an extremum point.
  3. Solve standard inequalities for the domain of the argument.
  4. Be able to prove in which functions a point on a graph is defined and continuous.

Attention! The search for a critical point of a function is possible only if there is a derivative of at least the second order, which is ensured by a high proportion of the presence of an extremum point.

Necessary condition for the extremum of the function

In order for an extremum to exist, it is important that there are both minimum points and maximum points. If this rule is observed only partially, then the condition for the existence of an extremum is violated.

Each function in any position must be differentiated in order to identify its new meanings. It is important to understand that the case when a point vanishes is not the main principle of finding a differentiable point.

A sharp extremum, as well as a function minimum, is an extremely important aspect of solving a mathematical problem using extreme values. In order to better understand this component, it is important to refer to the tabular values ​​for the assignment of the functional.

A complete exploration of meaning Plotting a Value
1. Determination of points of increase and decrease of values.

2. Finding break points, extremum and intersection with coordinate axes.

3. The process of determining changes in position on the chart.

4. Determination of the index and direction of convexity and convexity, taking into account the presence of asymptotes.

5. Creation of a summary table of the study in terms of determining its coordinates.

6. Finding intervals of increase and decrease of extreme and acute points.

7. Determination of the convexity and concavity of the curve.

8. Building a graph based on the study allows you to find a minimum or maximum.

 The main element, when it is necessary to work with extremums, is the exact construction of its graph.

School teachers do not often pay maximum attention to such an important aspect, which is a gross violation of the educational process.

The graph is built only on the basis of the results of the study of functional data, the definition of sharp extrema, as well as points on the graph.

Sharp extrema of the derivative of a function are displayed on a plot of exact values ​​using the standard procedure for determining asymptotes.

The maximum and minimum points of the function are accompanied by more complex plotting. This is due to a deeper need to work out the problem of a sharp extremum.

It is also necessary to find the derivative of a complex and simple function, since this is one of the most important concepts in the problem of extremum.

Functional extremum

In order to find the above value, you must adhere to the following rules:

  • determine the necessary condition for the extremal ratio;
  • take into account the sufficient condition of the extreme points on the graph;
  • carry out the calculation of an acute extremum.

There are also concepts such as weak minimum and strong minimum. This must be taken into account when determining the extremum and its exact calculation. At the same time, sharp functionality is the search and creation of all the necessary conditions for working with the function graph.

This is a rather interesting section of mathematics that absolutely all graduate students and students face. However, not everyone likes matan. Some fail to understand even basic things like the seemingly standard function study. This article aims to correct this oversight. Want to learn more about function analysis? Would you like to know what extremum points are and how to find them? Then this article is for you.

Investigation of the graph of a function

To begin with, it is worth understanding why it is necessary to analyze the chart at all. There are simple functions that are easy to draw. A striking example of such a function is the parabola. It's not hard to draw her chart. All that is needed is, using a simple transformation, to find the numbers at which the function takes the value 0. And in principle, this is all you need to know in order to draw a parabola graph.

But what if the function we need to graph is much more complicated? Since the properties of complex functions are rather non-obvious, it is necessary to carry out a whole analysis. Only then can the function be represented graphically. How to do it? You can find the answer to this question in this article.

Function analysis plan

The first thing to do is to conduct a superficial study of the function, during which we will find the domain of definition. So, let's start in order. The domain of definition is the set of those values ​​by which the function is defined. Simply put, these are the numbers that can be used in the function instead of x. In order to determine the scope, you just need to look at the entry. For example, it is obvious that the function y (x) \u003d x 3 + x 2 - x + 43 has a domain of definition - the set of real numbers. Well, with a function like (x 2 - 2x) / x, everything is a little different. Since the number in the denominator should not be equal to 0, then the domain of this function will be all real numbers, except for zero.

Next, you need to find the so-called zeros of the function. These are the values ​​of the argument for which the entire function takes the value zero. To do this, it is necessary to equate the function to zero, consider it in detail and perform some transformations. Let us take the already familiar function y(x) = (x 2 - 2x)/x. From the school course, we know that a fraction is 0 when the numerator is zero. Therefore, we discard the denominator and start working with the numerator, equating it to zero. We get x 2 - 2x \u003d 0 and take x out of brackets. Hence x (x - 2) \u003d 0. As a result, we get that our function is equal to zero when x is equal to 0 or 2.

During the study of the graph of a function, many are faced with a problem in the form of extremum points. And it's weird. After all, extremes are a rather simple topic. Don't believe? See for yourself by reading this part of the article, in which we will talk about the minimum and maximum points.

To begin with, it is worth understanding what an extremum is. An extremum is the limit value that a function reaches on a graph. From this it turns out that there are two extreme values ​​- a maximum and a minimum. For clarity, you can look at the picture above. On the investigated area, point -1 is the maximum of the function y (x) \u003d x 5 - 5x, and point 1, respectively, is the minimum.

Also, do not confuse concepts with each other. The extremum points of a function are those arguments at which the given function acquires extreme values. In turn, the extremum is the value of the minima and maxima of the function. For example, consider the figure above again. -1 and 1 are the extremum points of the function, and 4 and -4 are the extremums themselves.

Finding extremum points

But how do you find the extremum points of a function? Everything is pretty simple. The first thing to do is to find the derivative of the equation. Let's say we got the task: "Find the extremum points of the function y (x), x is the argument. For clarity, let's take the function y (x) \u003d x 3 + 2x 2 + x + 54. Let's differentiate and get the following equation: 3x 2 + 4x + 1. As a result, we got the standard quadratic equation. All that needs to be done is to equate it to zero and find the roots. Since the discriminant is greater than zero (D \u003d 16 - 12 \u003d 4), this equation is determined by two roots. We find them and get two values: 1/3 and -1. These will be the extremum points of the function. However, how can you still determine who is who? Which point is the maximum and which is the minimum? To do this, you need to take a neighboring point and find out its value. For example , take the number -2, which is to the left of the coordinate line from -1. We substitute this value in our equation y (-2) = 12 - 8 + 1 = 5. As a result, we got a positive number. This means that on the interval from 1/3 to -1 the function increases, which in turn means that on the intervals from minus infinity to 1/3 and from -1 to plus infinity the function decreases. Thus, we can conclude that the number 1/3 is the minimum point of the function on the investigated interval, and -1 is the maximum point.

It is also worth noting that the USE requires not only to find extremum points, but also to perform some kind of operation with them (add, multiply, etc.). It is for this reason that it is worth paying special attention to the conditions of the problem. After all, due to inattention, you can lose points.

The extremum point of a function is the point in the function's domain where the value of the function takes on a minimum or maximum value. The function values ​​at these points are called extrema (minimum and maximum) of the function.

Definition. Dot x1 function scope f(x) is called maximum point of the function , if the value of the function at this point is greater than the values ​​of the function at points close enough to it, located to the right and left of it (that is, the inequality f(x0 ) > f(x 0 + Δ x) x1 maximum.

Definition. Dot x2 function scope f(x) is called minimum point of the function, if the value of the function at this point is less than the values ​​of the function at points close enough to it, located to the right and left of it (that is, the inequality f(x0 ) < f(x 0 + Δ x) ). In this case, the function is said to have at the point x2 minimum.

Let's say the point x1 - maximum point of the function f(x) . Then in the interval up to x1 function increases, so the derivative of the function is greater than zero ( f "(x) > 0 ), and in the interval after x1 the function is decreasing, so function derivative less than zero ( f "(x) < 0 ). Тогда в точке x1

Let us also assume that the point x2 - minimum point of the function f(x) . Then in the interval up to x2 the function is decreasing and the derivative of the function is less than zero ( f "(x) < 0 ), а в интервале после x2 the function is increasing and the derivative of the function is greater than zero ( f "(x) > 0 ). In this case also at the point x2 the derivative of the function is zero or does not exist.

Fermat's theorem (a necessary criterion for the existence of an extremum of a function). If point x0 - extremum point of the function f(x) , then at this point the derivative of the function is equal to zero ( f "(x) = 0 ) or does not exist.

Definition. The points at which the derivative of a function is equal to zero or does not exist are called critical points .

Example 1 Let's consider a function.

At the point x= 0 the derivative of the function is equal to zero, therefore, the point x= 0 is the critical point. However, as can be seen on the graph of the function, it increases in the entire domain of definition, so the point x= 0 is not an extremum point of this function.

Thus, the conditions that the derivative of a function at a point is equal to zero or does not exist are necessary conditions for an extremum, but not sufficient, since other examples of functions can be given for which these conditions are satisfied, but the function does not have an extremum at the corresponding point. That's why must have sufficient indications, which make it possible to judge whether there is an extremum at a particular critical point and which one - a maximum or a minimum.

Theorem (the first sufficient criterion for the existence of an extremum of a function). Critical point x0 f(x) , if the derivative of the function changes sign when passing through this point, and if the sign changes from "plus" to "minus", then the maximum point, and if from "minus" to "plus", then the minimum point.

If near the point x0 , to the left and to the right of it, the derivative retains its sign, this means that the function either only decreases or only increases in some neighborhood of the point x0 . In this case, at the point x0 there is no extremum.

So, to determine the extremum points of the function, you need to do the following :

  1. Find the derivative of a function.
  2. Equate the derivative to zero and determine the critical points.
  3. Mentally or on paper, mark the critical points on the numerical axis and determine the signs of the derivative of the function in the intervals obtained. If the sign of the derivative changes from "plus" to "minus", then the critical point is the maximum point, and if from "minus" to "plus", then the critical point is the minimum point.
  4. Calculate the value of the function at the extremum points.

Example 2 Find extrema of a function .

Solution. Let's find the derivative of the function:

Equate the derivative to zero to find the critical points:

.

Since for any values ​​\u200b\u200bof "x" the denominator is not equal to zero, then we equate the numerator to zero:

Got one critical point x= 3 . We determine the sign of the derivative in the intervals delimited by this point:

in the range from minus infinity to 3 - minus sign, that is, the function decreases,

in the range from 3 to plus infinity - a plus sign, that is, the function increases.

That is, point x= 3 is the minimum point.

Find the value of the function at the minimum point:

Thus, the extremum point of the function is found: (3; 0) , and it is the minimum point.

Theorem (the second sufficient criterion for the existence of an extremum of a function). Critical point x0 is the extremum point of the function f(x) , if the second derivative of the function at this point is not equal to zero ( f ""(x) ≠ 0 ), moreover, if the second derivative is greater than zero ( f ""(x) > 0 ), then the maximum point, and if the second derivative is less than zero ( f ""(x) < 0 ), то точкой минимума.

Remark 1. If at a point x0 both the first and second derivatives vanish, then at this point it is impossible to judge the presence of an extremum on the basis of the second sufficient sign. In this case, you need to use the first sufficient criterion for the extremum of the function.

Remark 2. The second sufficient criterion for the extremum of a function is also inapplicable when the first derivative does not exist at the stationary point (then the second derivative does not exist either). In this case, it is also necessary to use the first sufficient criterion for the extremum of the function.

The local nature of the extrema of the function

From the above definitions it follows that the extremum of a function is of a local nature - this is the largest and smallest value of the function compared to the nearest values.

Suppose you consider your earnings in a time span of one year. If in May you earned 45,000 rubles, and in April 42,000 rubles, and in June 39,000 rubles, then the May earnings are the maximum of the earnings function compared to the nearest values. But in October you earned 71,000 rubles, in September 75,000 rubles, and in November 74,000 rubles, so the October earnings are the minimum of the earnings function compared to nearby values. And you can easily see that the maximum among the values ​​of April-May-June is less than the minimum of September-October-November.

Generally speaking, a function may have several extrema on an interval, and it may turn out that any minimum of the function is greater than any maximum. So, for the function shown in the figure above, .

That is, one should not think that the maximum and minimum of the function are, respectively, its maximum and minimum values ​​on the entire segment under consideration. At the point of maximum, the function has the greatest value only in comparison with those values ​​that it has at all points sufficiently close to the maximum point, and at the minimum point, the smallest value only in comparison with those values ​​that it has at all points sufficiently close to the minimum point.

Therefore, we can refine the above concept of extremum points of a function and call the minimum points local minimum points, and the maximum points - local maximum points.

We are looking for the extrema of the function together

Example 3

Solution. The function is defined and continuous on the whole number line. Its derivative also exists on the entire number line. Therefore, in this case, only those at which , i.e., serve as critical points. , whence and . Critical points and divide the entire domain of the function into three intervals of monotonicity: . We select one control point in each of them and find the sign of the derivative at this point.

For the interval, the reference point can be : we find . Taking a point in the interval, we get , and taking a point in the interval, we have . So, in the intervals and , and in the interval . According to the first sufficient sign of an extremum, there is no extremum at the point (since the derivative retains its sign in the interval ), and the function has a minimum at the point (since the derivative changes sign from minus to plus when passing through this point). Find the corresponding values ​​of the function: , and . In the interval, the function decreases, since in this interval , and in the interval it increases, since in this interval.

To clarify the construction of the graph, we find the points of intersection of it with the coordinate axes. When we obtain an equation whose roots and , i.e., two points (0; 0) and (4; 0) of the graph of the function are found. Using all the information received, we build a graph (see at the beginning of the example).

For self-checking during calculations, you can use online derivatives calculator .

Example 4 Find the extrema of the function and build its graph.

The domain of the function is the entire number line, except for the point, i.e. .

To shorten the study, we can use the fact that this function is even, since . Therefore, its graph is symmetrical about the axis Oy and the study can only be performed for the interval .

Finding the derivative and critical points of the function:

1) ;

2) ,

but the function suffers a break at this point, so it cannot be an extremum point.

Thus, the given function has two critical points: and . Taking into account the parity of the function, we check only the point by the second sufficient sign of the extremum. To do this, we find the second derivative and determine its sign at : we get . Since and , then is the minimum point of the function, while .

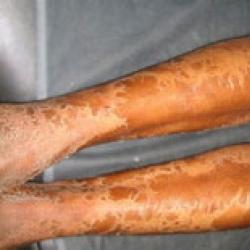
To get a more complete picture of the graph of the function, let's find out its behavior on the boundaries of the domain of definition:

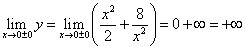
(here the symbol indicates the desire x to zero on the right, and x remains positive; similarly means aspiration x to zero on the left, and x remains negative). Thus, if , then . Next, we find

,

those. if , then .

The graph of the function has no points of intersection with the axes. The picture is at the beginning of the example.

For self-checking during calculations, you can use online derivatives calculator .

We continue to search for extremums of the function together

Example 8 Find the extrema of the function .

Solution. Find the domain of the function. Since the inequality must hold, we obtain from .

Let's find the first derivative of the function.

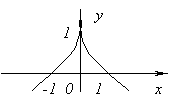
Consider the graph of a continuous function y=f(x) shown in the figure.

Function value at point x 1 will be greater than the values ​​of the function at all neighboring points both to the left and to the right of x 1 . In this case, the function is said to have at the point x 1 max. At the point x The 3 function obviously also has a maximum. If we consider the point x 2 , then the value of the function in it is less than all neighboring values. In this case, the function is said to have at the point x 2 minimum. Similarly for the point x 4 .

Function y=f(x) at the point x 0 has maximum, if the value of the function at this point is greater than its values ​​at all points of some interval containing the point x 0 , i.e. if there is such a neighborhood of the point x 0 , which is for everyone x≠x 0 , belonging to this neighborhood, we have the inequality f(x)<f(x 0 ) .

Function y=f(x) It has minimum at the point x 0 , if there is such a neighborhood of the point x 0 , what is for everyone x≠x 0 belonging to this neighborhood, we have the inequality f(x)>f(x0.

The points at which the function reaches its maximum and minimum are called extremum points, and the values ​​of the function at these points are the extrema of the function.

Let us pay attention to the fact that a function defined on a segment can reach its maximum and minimum only at points contained within the segment under consideration.

Note that if a function has a maximum at a point, this does not mean that at this point the function has the maximum value in the entire domain. In the figure discussed above, the function at the point x 1 has a maximum, although there are points at which the values ​​of the function are greater than at the point x 1 . In particular, f(x 1) < f(x 4) i.e. the minimum of the function is greater than the maximum. From the definition of the maximum, it only follows that this is the largest value of the function at points sufficiently close to the maximum point.

Theorem 1. (A necessary condition for the existence of an extremum.) If the differentiable function y=f(x) has at the point x= x 0 extremum, then its derivative at this point vanishes.

Proof. Let, for definiteness, at the point x 0 the function has a maximum. Then for sufficiently small increments Δ x we have f(x 0 + Δ x) 0 ) , i.e. But then

Passing in these inequalities to the limit as Δ x→ 0 and taking into account that the derivative f "(x 0) exists, and hence the limit on the left does not depend on how Δ x→ 0, we get: for Δ x → 0 – 0 f"(x 0) ≥ 0 and at Δ x → 0 + 0 f"(x 0) ≤ 0. Since f"(x 0) defines a number, then these two inequalities are compatible only if f"(x 0) = 0.

The proved theorem states that the maximum and minimum points can only be among those values ​​of the argument for which the derivative vanishes.

We have considered the case when a function has a derivative at all points of a certain segment. What happens when the derivative does not exist? Consider examples.

Examples.

  1. y=|x|.

    The function does not have a derivative at a point x=0 (at this point, the graph of the function does not have a definite tangent), but at this point the function has a minimum, since y(0)=0, and for all x≠ 0y > 0.

    2. The function has no derivative at x=0, since it goes to infinity when x=0. But at this point, the function has a maximum.

    The function has no derivative at x=0 because at x→0. At this point, the function has neither a maximum nor a minimum. Really, f(x)=0 and at x<0f(x)<0, а при x>0f(x)>0.

    Thus, from the given examples and the formulated theorem it is clear that the function can have an extremum only in two cases: 1) at the points where the derivative exists and is equal to zero; 2) at the point where the derivative does not exist.

    However, if at some point x 0 we know that f"(x 0 ) =0, then it cannot be concluded from this that at the point x 0 the function has an extremum.

    For example. .

    But point x=0 is not an extremum point, since to the left of this point the function values ​​are located below the axis Ox, and above on the right.

    Values ​​of an argument from the domain of a function, for which the derivative of the function vanishes or does not exist, are called critical points.


    It follows from the foregoing that the extremum points of a function are among the critical points, and, however, not every critical point is an extremum point. Therefore, to find the extremum of the function, you need to find all the critical points of the function, and then examine each of these points separately for maximum and minimum. For this, the following theorem serves.

    Theorem 2. (A sufficient condition for the existence of an extremum.) Let the function be continuous on some interval containing the critical point x 0 , and is differentiable at all points of this interval (except, perhaps, the point itself x 0). If, when passing from left to right through this point, the derivative changes sign from plus to minus, then at the point x = x 0 the function has a maximum. If, when passing through x 0 from left to right, the derivative changes sign from minus to plus, then the function has a minimum at this point.

    Thus, if

    Proof. Let us first assume that when passing through x 0, the derivative changes sign from plus to minus, i.e. for all x close to the point x 0 f "(x)> 0 for x< x 0 , f"(x)< 0 for x > x 0 . Let us apply the Lagrange theorem to the difference f(x) - f(x 0 ) = f "(c)(x- x 0), where c lies between x And x 0 .

    1. Let x< x 0 . Then c< x 0 and f "(c)> 0. That's why f "(c)(x-x 0)< 0 and, therefore,

      f(x) - f(x 0 )< 0, i.e. f(x)< f(x 0 ).

    2. Let x > x 0 . Then c>x 0 and f"(c)< 0. Means f "(c)(x-x 0)< 0. That's why f(x) - f(x 0 ) <0,т.е.f(x)< f(x 0 ) .

    Thus, for all values x close enough to x 0 f(x)< f(x 0 ) . And this means that at the point x 0 the function has a maximum.

    The second part of the minimum theorem is proved similarly.

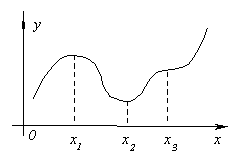
    Let us illustrate the meaning of this theorem in the figure. Let f"(x 1 ) =0 and for any x, close enough to x 1 , the inequalities

    f"(x)< 0 at x< x 1 , f "(x)> 0 at x > x 1 .

    Then to the left of the point x 1 the function is increasing, and decreasing on the right, therefore, when x = x 1 function goes from increasing to decreasing, that is, it has a maximum.

    Similarly, one can consider the points x 2 and x 3 .


    Schematically, all of the above can be depicted in the picture:

    The rule for studying the function y=f(x) for an extremum

    1. Find the scope of a function f(x).
    2. Find the first derivative of a function f"(x).
    3. Determine critical points, for this:
      1. find the real roots of the equation f"(x)=0;
      2. find all values x under which the derivative f"(x) does not exist.
    4. Determine the sign of the derivative to the left and right of the critical point. Since the sign of the derivative remains constant between two critical points, it suffices to determine the sign of the derivative at any one point to the left and at one point to the right of the critical point.
    5. Calculate the value of the function at the extremum points.

    Examples. Explore functions for minimum and maximum.


    THE GREATEST AND MINIMUM FUNCTION VALUES ON THE INTERCEPT

    the greatest the value of a function on a segment is the largest of all its values ​​on this segment, and least is the smallest of all its values.

    Consider the function y=f(x) continuous on the segment [ a, b]. As is known, such a function reaches its maximum and minimum values, either on the boundary of the segment, or inside it. If the maximum or minimum value of the function is reached at the internal point of the segment, then this value is the maximum or minimum of the function, that is, it is reached at critical points.

    Thus, we get the following the rule for finding the largest and smallest values ​​of a function on the segment [ a, b] :

    1. Find all critical points of a function in the interval ( a, b) and calculate the function values ​​at these points.
    2. Calculate the values ​​of the function at the ends of the segment for x=a, x=b.
    3. Of all the obtained values, choose the largest and smallest.